http://www.phys.unsw.edu.au/jw/dBNoFlash.html
What is a decibel?
And what are the different types of decibel measurement: dB, dBA, dBC, dBi? How are they related to loudness? (A related page allows you to measure your hearing response and to compare with standard hearing curves.)
* Definition and examples
* Sound files to show the size of a decibel
* Standard reference levels ("absolute" sound level)
* Logarithmic response, psychophysical measures, sones and phons
* dBi and radiation
Definition and examples
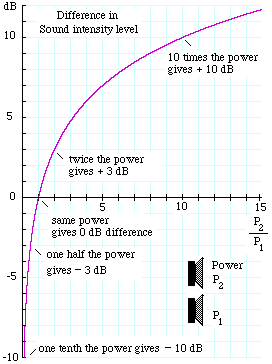
The decibel (dB) is used to measure sound level, but it is also widely used in electronics, signals and communication. The dB is a logarithmic unit used to describe a ratio. The ratio may be power, sound pressure, voltage or intensity or several other things. Later on we relate dB to the phon and the sone (other units related to loudness). But first, to get a taste for logarithmic units, let's look at some numbers:
For instance, suppose we have two loudspeakers, the first playing a sound with power P1, and another playing a louder version of the same sound with power P2, but everything else (how far away, frequency) kept the same.
The difference in decibels between the two is defined to be
10 log (P2/P1) dB where the log is to base 10.
If the second produces twice as much power than the first, the difference in dB is
10 log (P2/P1) = 10 log 2 = 3 dB.
If the second had 10 times the power of the first, the difference in dB would be
10 log (P2/P1)= 10 log 10 = 10 dB.
If the second had a million times the power of the first, the difference in dB would be
10 log (P2/P1) = 10 log 1000000 = 60 dB.
This example shows one feature of decibel scales that is useful in discussing sound: they can describe very big ratios using numbers of modest size. But note that the decibel describes a ratio: so far we have not said what power either of the speakers radiates, only the ratio of powers.
graph of dB scale
Plot of 10 log (P2/P1)
Sound pressure, sound level and dB. Sound is usually measured with microphones and they respond (approximately) proportionally to the sound pressure, p. Now the power in a sound wave, all else equal, goes as the square of the pressure. (Similarly, electrical power goes as the square of the voltage.) The log of the square of x is just 2 log x, so this introduces a factor of 2 when we convert to decibels for pressures. The difference in sound pressure level between two sounds with p1 and p2 is therefore:
20 log (p2/p1) dB = 10 log (p22/p12) dB = 10 log (P2/P1) dB where again the log is to base 10.
Sound files to show the size of a decibel
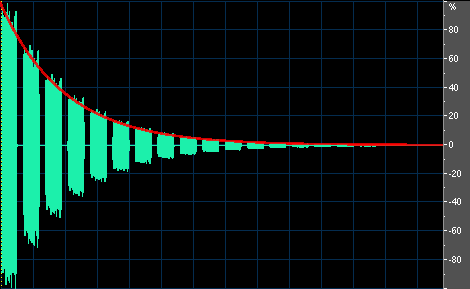
What happens when you halve the sound power? The log of 2 is 0.3, so the log of 1/2 is -0.3. So, if you halve the power, you reduce the power and the sound level by 3 dB. Halve it again (down to 1/4 of the original power) and you reduce the level by another 3 dB. That is exactly what we have done in the first graphic and sound file below.
wav mp3 Broadband noise decreasing by 3 dB steps.
The first sample of sound is white noise (a mix of all audible frequencies, just as white light is a mix of all visible frequencies). The second sample is the same noise, with the voltage reduced by a factor of the square root of 2. 2-0.5 is approximately 0.7, so -3 dB corresponds to reducing the voltage or the pressure to 70% of its original value. The green line shows the voltage as a function of time. The red line shows a continuous exponential decay with time. Note that the voltage falls by 50% for every second sample.
Note, too, that a doubling of the power does not make a huge difference to the loudness. We'll discuss this further below, but it's a useful thing to remember when choosing sound reproduction equipment.
Sound files and graph by John Tann and George Hatsidimitris.
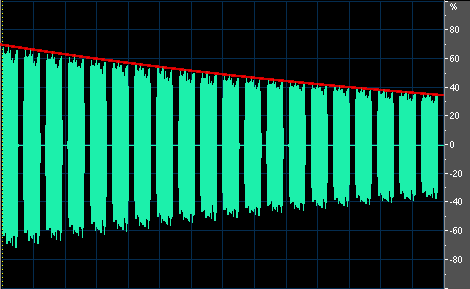
How big is a decibel? In the next series, successive samples are reduced by just one decibel.

wav mp3 Broadband noise decreasing by 1 dB steps.
One decibel is close to the Just Noticeable Difference (JND) for sound level. As you listen to these files, you will notice that the last is quieter than the first, but it is rather less clear to the ear that the second of any pair is quieter than its predecessor. 10*log10(1.26) = 1, so to increase the sound level by 1 dB, the power must be increased by 26%, or the voltage by 12%.
What if the difference is less than a decibel? Sound levels are rarely given with decimal places. The reason is that sound levels that differ by less than 1 dB are hard to distinguish, as the next example shows.
wav mp3 Broadband noise decreasing by 0.3 dB steps.
You may notice that the last is quieter than the first, but it is difficult to notice the difference between successive pairs. 10*log10(1.07) = 0.3, so to increase the sound level by 0.3 dB, the power must be increased by 7%, or the voltage by 3.5%.
Standard reference levels ("absolute" sound level)
When the decibel is used to give the sound level for a single sound rather than a ratio, then a reference level must be chosen. For sound intensity, the reference level (for air) is usually chosen as 20 micropascals, or 0.02 mPa. (This is very low: it is 2 ten billionths of an atmosphere. Nevertheless, this is about the limit of sensitivity of the human ear, in its most sensitive range of frequency. Usually this sensitivity is only found in rather young people or in people who have not been exposed to loud music or other loud noises. Personal music systems with in-ear speakers ('walkmans') are capable of very high sound levels in the ear, and are believed by some to be responsible for much of the hearing loss in young adults in developed countries.)
So if you read of a sound intensity level of 86 dB, it means that
20 log (p2/p1) = 86 dB
where p1 is the sound pressure of the reference level, and p2 that of the sound in question. Divide both sides by 20:
log (p2/p1) = 4.3
p2/p1 = 104.3
4 is the log of 10 thousand, 0.3 is the log of 2, so this sound has a sound pressure 20 thousand times greater than that of the reference level (p2/p1 = 20,000). 86 dB is a loud but not dangerous level of sound, if it is not maintained for very long.
What does 0 dB mean? This level occurs when the measured intensity is equal to the reference level. i.e., it is the sound level corresponding to 0.02 mPa. In this case we have
sound level = 20 log (pmeasured/preference) = 20 log 1 = 0 dB
So 0 dB does not mean no sound, it means a sound level where the sound pressure is equal to that of the reference level. This is a small pressure, but not zero. It is also possible to have negative sound levels: - 20 dB would mean a sound with pressure 10 times smaller than the reference pressure, ie 2 micropascals.
Not all sound pressures are equally loud. This is because the human ear does not respond equally to all frequencies: we are much more sensitive to sounds in the frequency range about 1 kHz to 4 kHz (1000 to 4000 vibrations per second) than to very low or high frequency sounds. For this reason, sound meters are usually fitted with a filter whose response to frequency is a bit like that of the human ear. (More about these filters below.) If the "A weighting filter" is used, the sound pressure level is given in units of dB(A). Sound pressure level on the dB(A) scale is easy to measure and is therefore widely used. It is still different from loudness, however, because the filter does not respond in quite the same way as the ear. To determine the loudness of a sound, one needs to consult some (idealised) curves representing the frequency response of the human ear. (Alternatively, you can measure your own hearing response.)